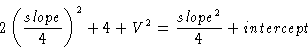

Next: About this document ...
Legendre Transformations (II, An example)
Consider a system whose energy has the form:
E = 2S2 + 4 + V2 = E(S,V)
where E is an explicit function of S and V (as required
in elementary thermo).
At constant V, we have
Figure 1:
E(S) is parabolic in S, as is A(T) in T, via the Legendre Transformations
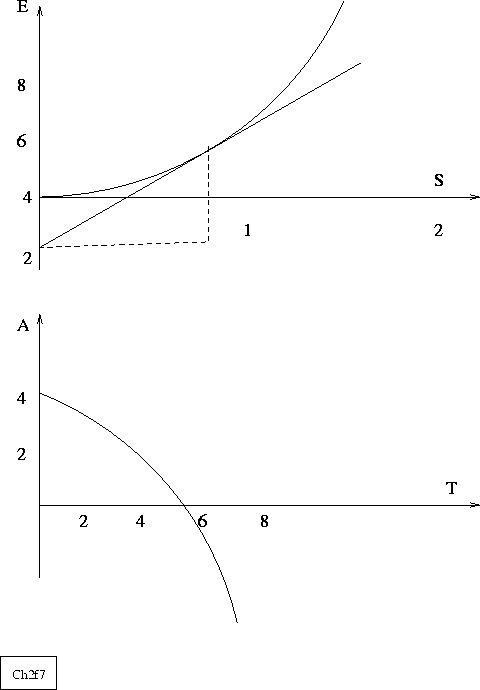 |
We wish to perform a Legendre Transformation on E(S,V) to change
the S variable to something else (it's going to be the temperature).
We wish
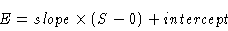 |
(1) |
Since the slope is 4S (see above) we have S=slope/4, so that,
substituting into equation 1 we have
so that
which is, substituting (slope/4) for S on the left hand side
which is an equation for the intercept as a function of the slope
(and V, which is being carried along here):
If we call the intercept A, the Helmholtz Free Energy, and the slope we
call T, the temperature, then we have
which is the desired result.
That is, we have started with a function of S and V and ended up with a function
of T and V.
Just to prove that we know what we're doing, we will do it again, this time
transforming A(T,V) into E(S,V).
We start with desiring a representation of A(T,V) of the form:
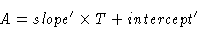 |
(2) |
Then
i.e.,
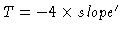 .
Substituting for T in equation 2
.
Substituting for T in equation 2
which is, substituting on the l.h.s.
which is, again substituting for T,
which rearranges to
i.e.
which is what we started with (sorry about the sentence construction).


Next: About this document ...
1998-04-07