Entropy Maximum and Energy Minimum

Although all elementary physical chemistry texts deal with entropy increases and spontaneity for isolated systems, it is not commonly understood that the energy decreasing at constant entropy, the other part of the minimax scheme for understanding spontaneity, also represents spontaneity. In a discussion of the Helmholtz free energy (A = E - TS), the statement is made that A includes the effect of both E dropping and S increasing, resulting in the sign of A being an index of spontaneity. We re-introduce the idea that an irreversible energy decrease at constant entropy is a spontaneous process by use of an explicit example.

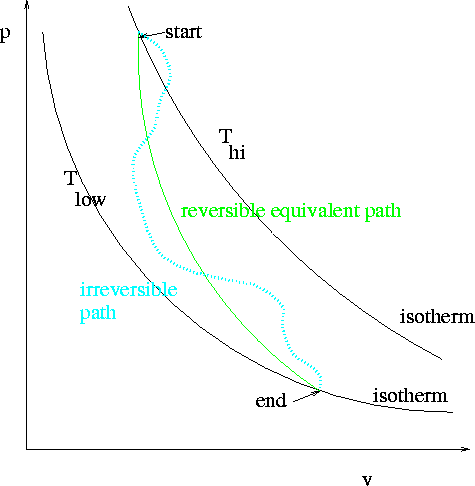
Consider a mole of ideal gas at T_start , p_start, and V_start. We keep the gas in a traditional piston cylinder arrangement. Now we place stops (chocks) on the piston to prevent its upward motion and then we reduce the pressure on the outside to p_end. The chocks provide the (extra) equivalent force so that initially, the gas is still being subject to a pressure of p_start. Next, we plunge the piston cylinder apparatus into a large temperature bath (standard disclaimers apply), whose temperature will remain constant at T_low, while simultaneously removing the chocks, so that the gas suddenly "sees" only p_end.

There is no question about what will happen next. The gas will expand and cool, irreversibly. It will do so spontaneously. T_low is lower than T_hi (by definition).

We are conducting an irreversible expansion of an ideal gas against a constant (lower pressure) while simultaneously cooling it. At the end of the expansion/cooling, T_low, since we used a large heat bath, p_end, i.e., the chocks having been removed, the only pressure is the final one, and the final volume is computable from the ideal gas law.

If we were smart enough to set the conditions so that the final equilibrium position of the piston, once attained, corresponded to increasing the volume to a value which places us where:

$$p_{end} \left ( V_{end} \right )^\gamma= p_{start} \left ( V_{start} \right )^\gamma$$

then we have created a situation in which the entropy change of the gas is exactly zero .

 

Figure 1: An Irreversible Expansion Against a Constant Pressure with Accompanying Decrease in Energy

(Notice that the "irreversible path" is dotted on the figure, since one can not show such a path correctly on an equilibrium diagram such as this.) The reversible equivalent path which gets us from our initial to our final situation corresponds to an adiabatic, i.e., a path for which there is no entropy change. We have illustrated a spontaneous irreversible process in which the entropy of the system stays the same, but the energy ... drops! This is because we agreed that T_end < T_start.

$$\Delta E_{system} = C_V \left ( T_{end} - T_{start} \right )$$

will be negative.

It is hard to imagine that the process illustrated could, spontaneously and by its own devising, proceed backwards from our final to our initial state. Thus we have associated a decrease in energy at constant entropy with spontaneity in the same way we normally associate an increase in entropy at constant energy with that selfsame spontaneity.
That function which combines both the entropy increase and the energy decrease associated with spontaneity (A) has been chosen (E-TS) so that if either E decreases or S increases (with the other constant) the function drops in value. Further, this function shows us that entropy increases with contravening energy increases can lead to non-spontaneous processes, and entropy decreases coupled with energy decreases can also lead to spontaneity.